12 ‘Open Middle’ math problems for a fun challenge

If you and your child are looking for a fun and challenging family activity, try these Open Middle math problems! Each one has a beginning and an end, but it’s up to each child’s thinking to do the work in the middle. Instead of giving the answers, just ask your child questions like these— “How did you solve it?,”
“Is there a different way?,” and “Can you explain your thinking?”

Kindergarten. Using the digits 1 to 5— no more than once for each number sentence— fill in the boxes to create two or more true number sentences.
1st Grade. Using the digits 1 to 9— no more than once for each number— place a digit in each box to create a true statement.
2nd Grade. Using only the digits 1 to 7— no more than once for each number— fill in the boxes to create a true equation.
3rd Grade. Using the digits 1 to 9— no more than once for each number— fill in the blanks to make the following problem true:
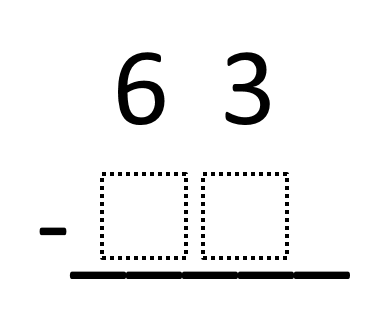
Sarah planted __ __ carrots in her garden. She planted them in __ rows. Each row had __ carrots.
4th Grade. Fill in the boxes so that you would need to regroup when you subtract. Make sure that your number is less than 63.
5th Grade. Using the digits 1 to 9— no more than once for each number— fill in the boxes to make the product as close to 7,000 as possible.
6th Grade. Using the digits 1 to 9— no more than once for each number— fill in the boxes to make three decimals whose sum is as close to 1 as possible.
7th Grade. Using the digits 1 to 6— no more than once for each number— fill in the boxes so that top two equations are equal and the bottom equation has the greatest value.
Algebra I. Using the digits 0 to 9— no more than once for each number— fill in the boxes to create an inequality whose solution set is x < -1/2.
Algebra I. Make a table with three points in the same line with
1) a slope not equal to zero; and
2) and the y-intercept is not a whole number. Write the equation for the line.
Geometry. For the figure, list three sequences of transformations that take pre-image ABCD to image A’B’C’D’.
Geometry. Given triangle ABC with vertices (-8, 2), (-2, 2), and (-2, 8), create triangle DEF in quadrant one that uses a translation, rotation, and reflection (in any order) to take that triangle to triangle ABC and show congruence.

We’ll be sharing more tips so watch for the next installment of DLD Daily Dozen!